- Light Source
- Plane Wave
- Circular, 100 mm radius
- Wavelength: 633 nm
- Power 1 W
- Unpolarized
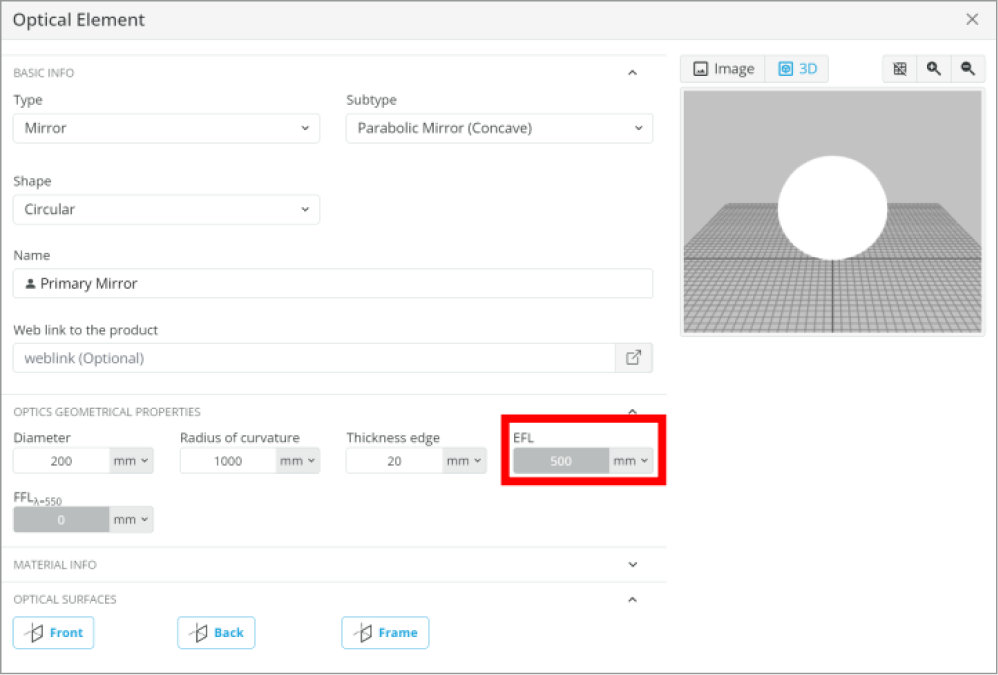
- Primary Mirror, Parabolic
- Circular, 200 mm diameter
- Focal Length: 500 mm
- Ideal Reflective Coating
- Flat Mirror
- Circular, 50 mm diameter
- Ideal Reflective Coating
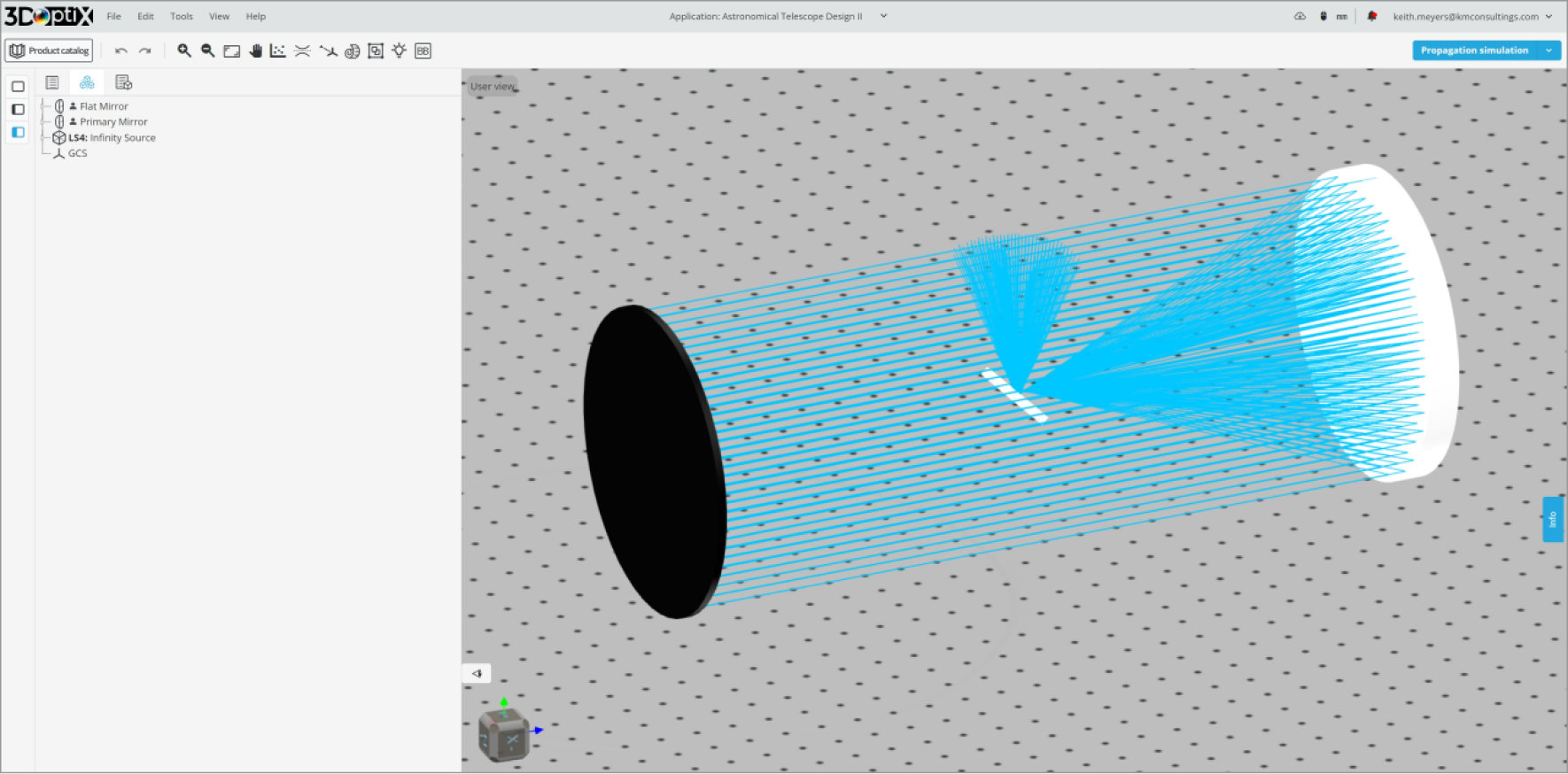
- Wide field of view for observing larger astronomical objects
- Inverted image
- Good light-gathering characteristics
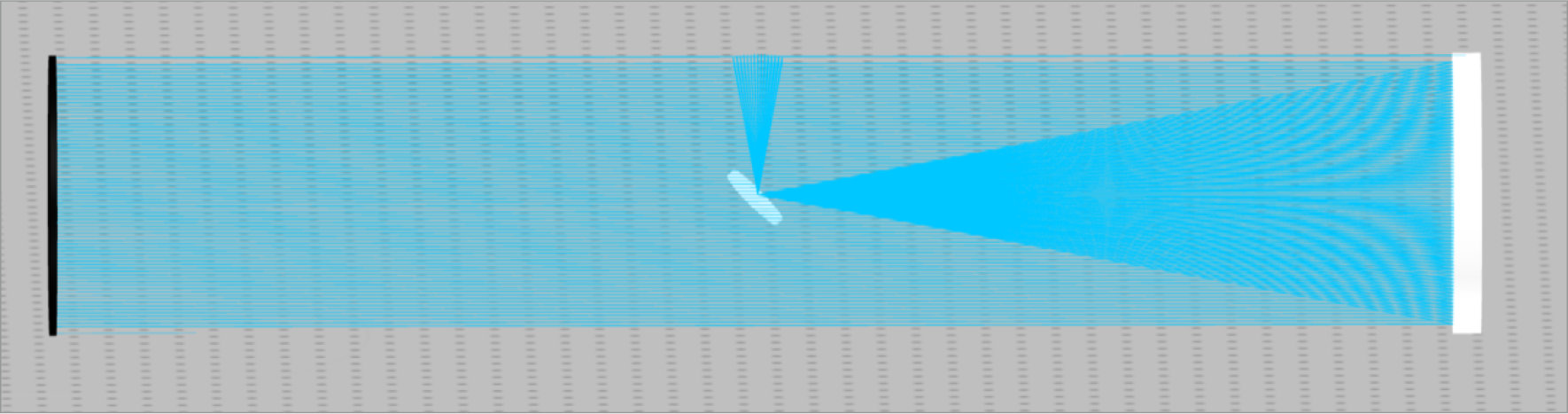
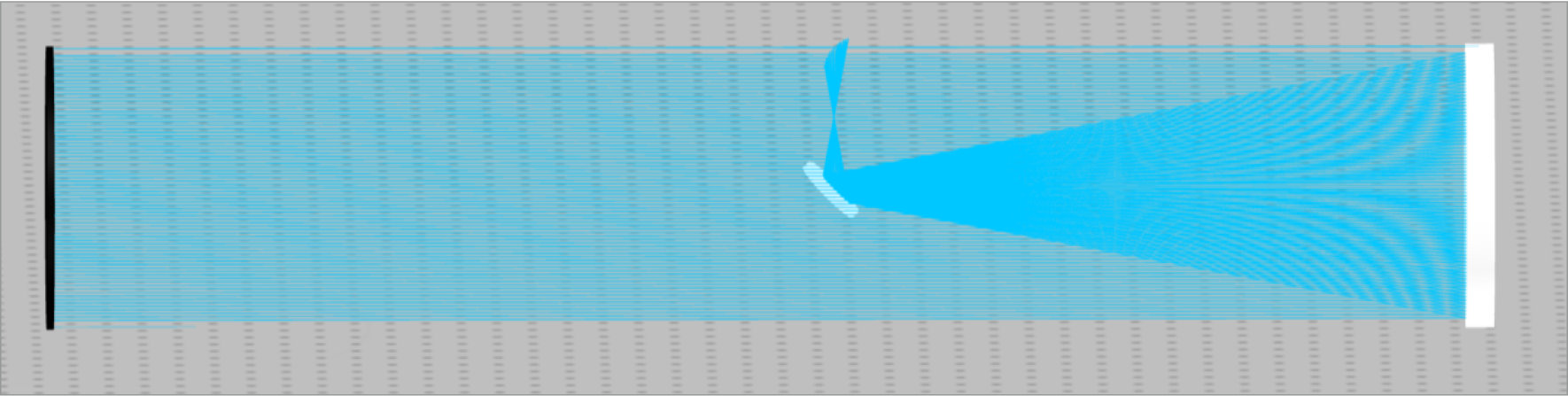
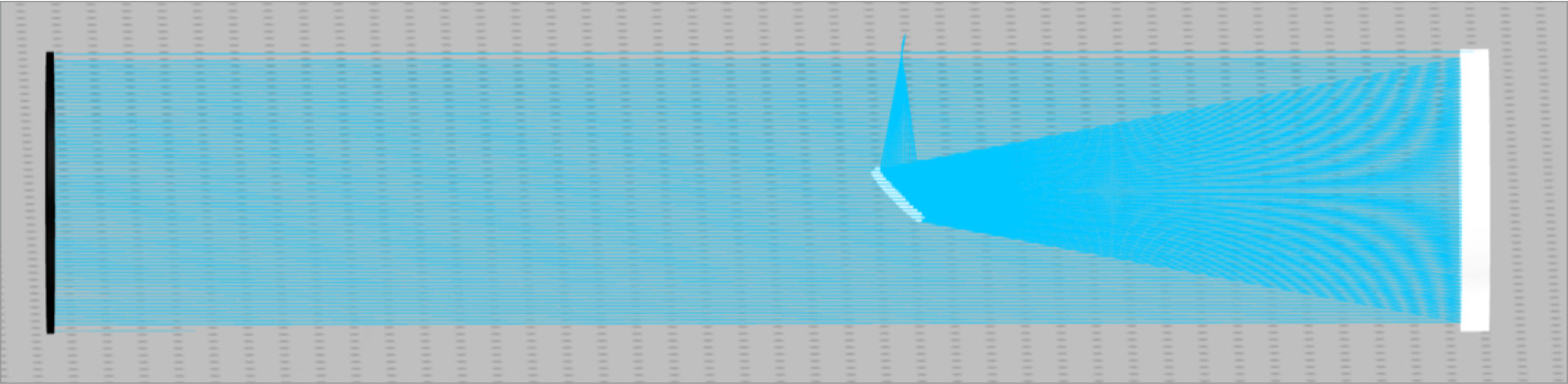
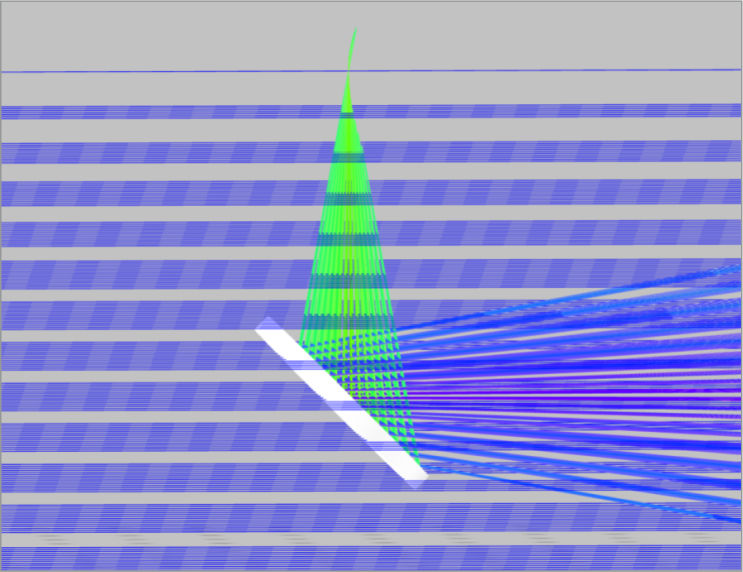
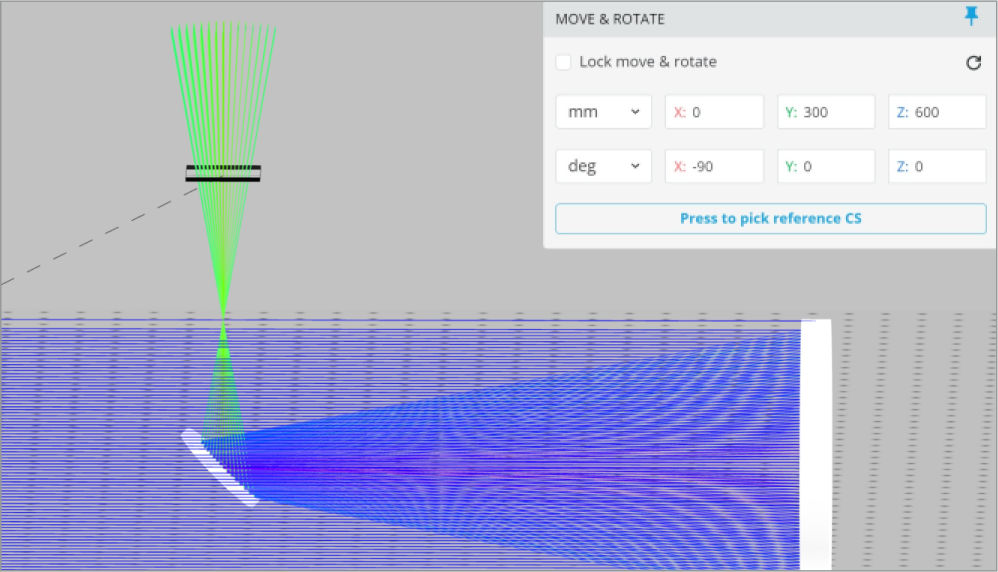
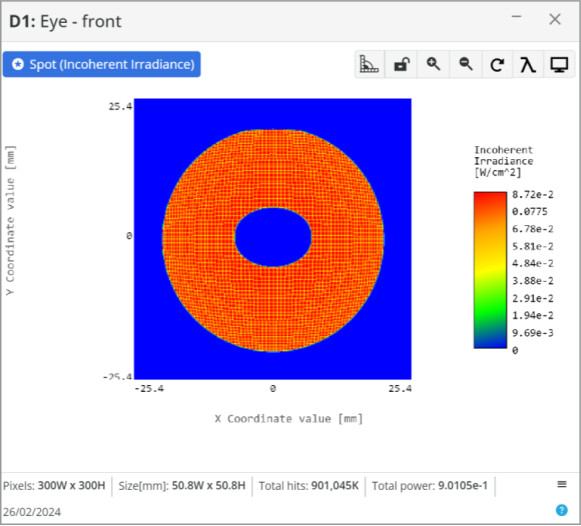
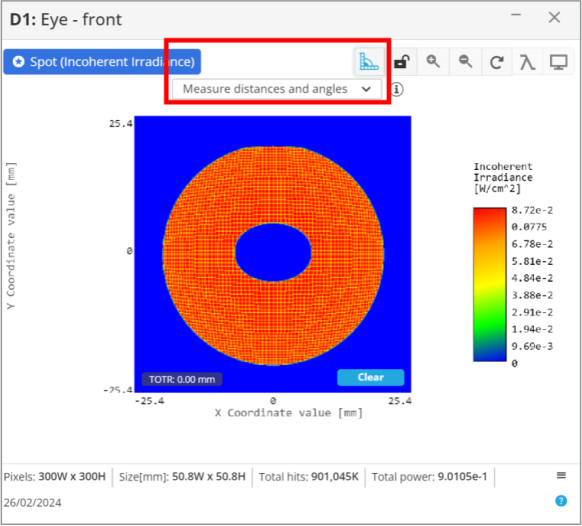
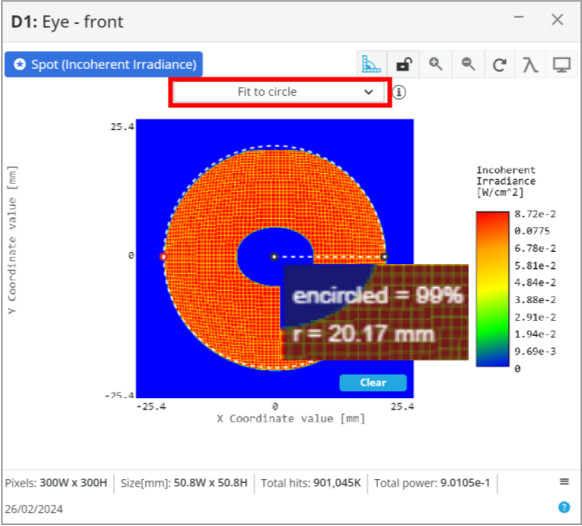
Now that the eyepiece lens diameter is determined, the focal length is the final parameter needed. We can place another detector approximately near the focus in the 3D layout to measure this, but it is easier to just use the focal length of the primary mirror and do a quick calculation.
The primary mirror focal length is 500 mm, and the primary to secondary mirror separation is 400 mm. The distance from the secondary mirror to the detector we placed is 200 mm. Using these numbers, we know a lens with a focal length of 100 mm needs to be placed where the detector is currently positioned
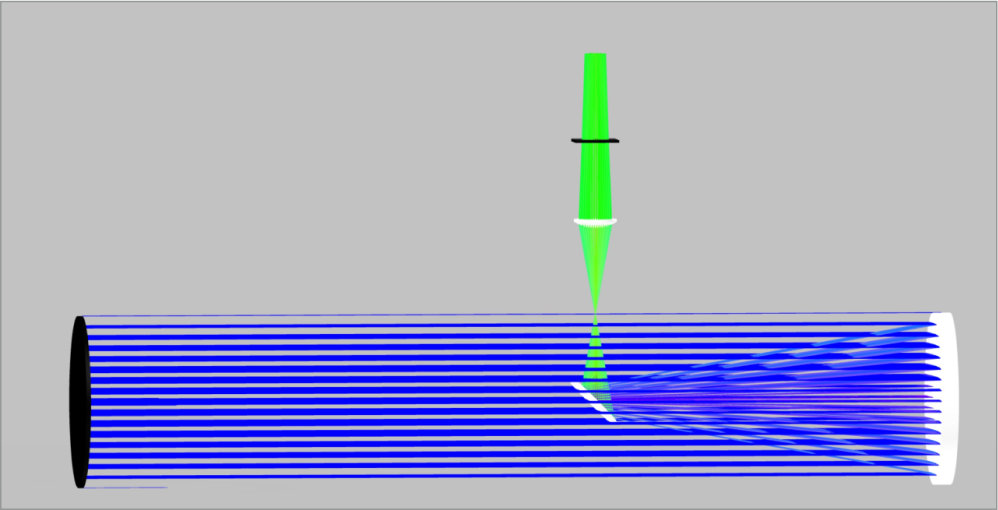
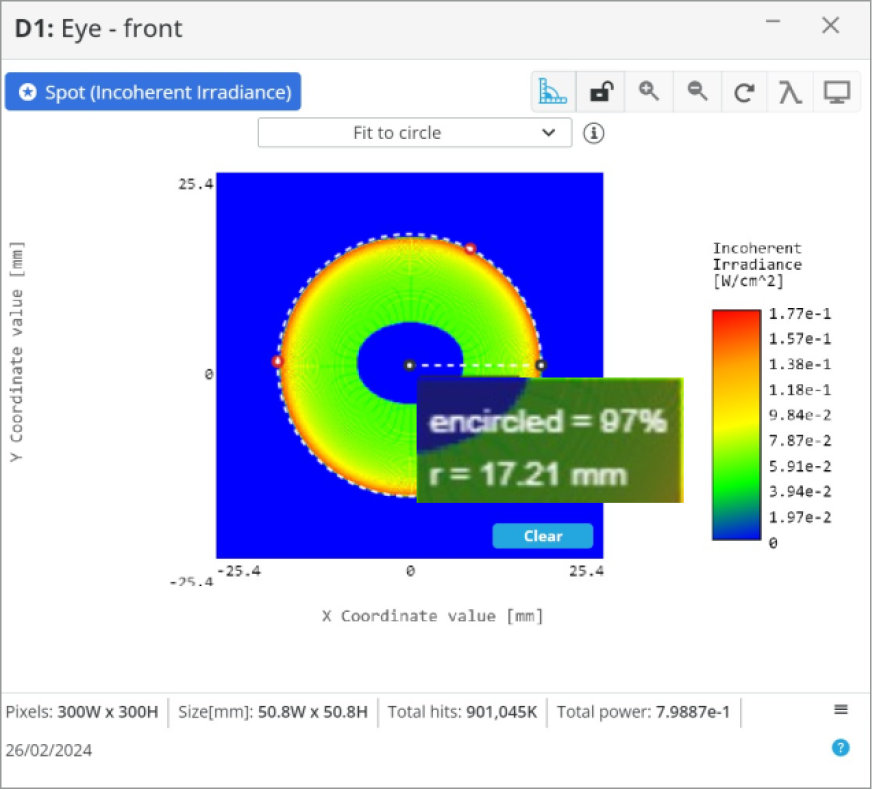
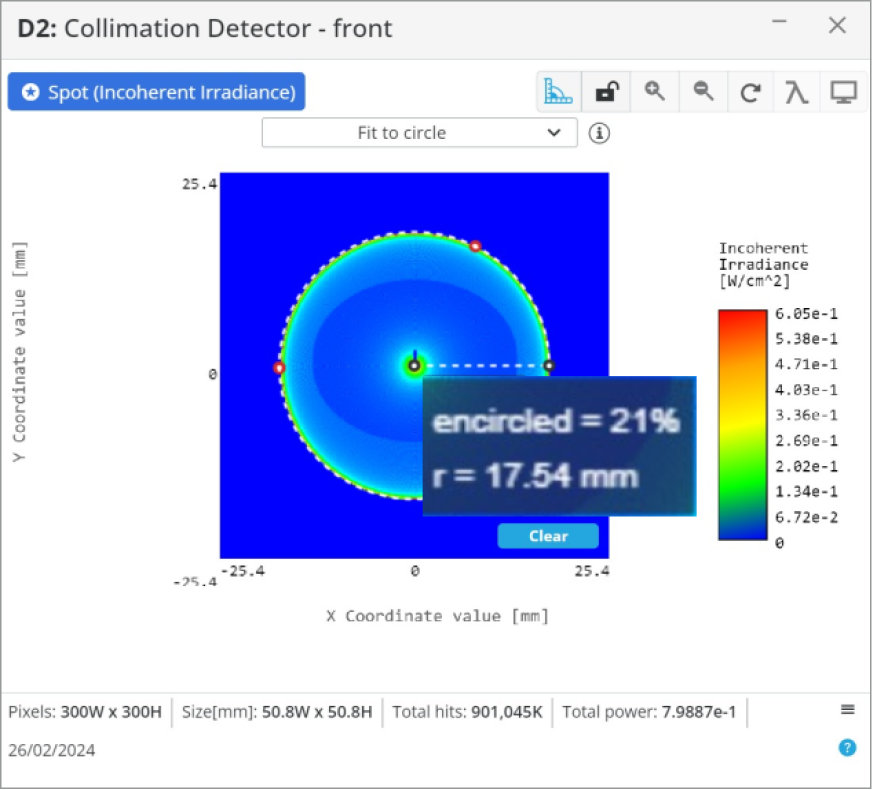
The last item we want to introduce is a 25 mm paraxial lens that simulates a camera lens and places the detector at the paraxial lens focus to simulate the focal plane array.
One note, if we wanted to create the telescope for viewing with an eye, we would want to use a smaller diameter and short focal length lens to create a smaller spot size. Otherwise, with the 40 mm spot size we currently have, the iris of the eye would block most of the light as it is only ~10 mm in diameter.
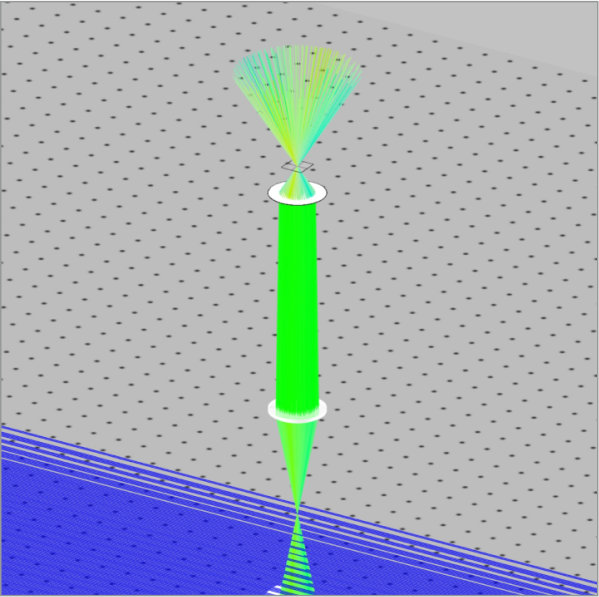
- System Magnification: M = \frac{f_{System}}{f_{eyepiece}} = \frac{500}{100} = 5x
Now that we have a baseline design of a Newtonian reflector telescope, we can further change and test the optical configuration. Using known equations that are used for designing these types of astronomical telescopes we can further optimize and analyze the system. The analysis features we have access to will be very helpful in further efforts.





